Dissecting a mystery pitch
On April 29, 2011, during the top of the fifth inning of a Toronto at New York game, Yankee pitcher Freddy Garcia threw a split-fingered fastball to the Jay’s Juan Rivera. A video of the pitch was captured by YES-MO, the high-speed video camera from the Yankees television broadcast, with sufficient resolution to allow both the spin rate and the rotation axis of the ball to be determined with reasonable precision.
Before reading on, you should take a look at the video, paying attention to the following details:
- The ball makes a bit over five complete rotations on its sojourn to home plate.
- The rotation axis is such that the rear of the ball, as seen in the video, is turning down and to the left
- The ball has a movement that is down and to the pitcher’s left
 |
| Juan Rivera swings and misses at Freddie Garcia’s splitter. This image is a frame capture from the YES-MO video. |
So, does the behavior of this pitch make any sense to you? If you answer NO, then you are among the vast majority of those who have studied this pitch, including both baseball analysts and scientists. So, why is this pitch so unusual? Or, said slightly differently, what would we expect the movement of the pitch to be given what we observe about the spin?
Let’s digress for a moment. First, what do we mean by “movement?” Movement is quite simply the deviation of the ball from a straight-line trajectory. For there to be movement, there has to be a force exerted on the ball that is transverse to its direction of motion. For most baseball pitches, there are two sources of transverse force: gravity and the Magnus force. Let’s talk about both.
Gravity is the dominant of these forces and is intuitively understood by most of us: It pulls everything downward. The Magnus force is the aerodynamic force on a spinning baseball. Its magnitude is roughly proportional to the rotation rate of the baseball: The faster it spins, the larger the force.
What about the direction? Well, the easiest way to remember the direction of the Magnus force is that it is always in the direction that the leading edge of the ball is turning. The leading edge is the side of the ball seen by the batter.
As an example, a typical four-seam fastball is thrown with backspin, so the leading edge of the ball is turning up, and the Magnus force is upward, opposing gravity. The force of gravity is still larger than the Magnus force, so the net vertical movement is downward but less than it would have been with gravity alone.
Likewise, a “12-to-6” curveball has topspin, such that the leading edge is turning downward; consequently, the downward movement is greater than it would have been with gravity alone.
A two-seam fastball has it rotation axis oriented slightly differently so that the baseball has a slight sidespin component to it. In fact, the Garcia pitch, despite being a splitter, is thrown in the two-seam orientation. Since the spin axis is tilted, there is reduced backspin (so that the ball drops more than a typical four-seamer).
What about the side spin? As you can observe from the video, the back of the ball—the side we and the pitcher see—is turning down and to the left. That means the leading edge of the ball—the side seen by the batter—is turning up and to the pitcher’s right. That means the expected movement from the Magnus force is up and to the pitcher’s right.
The fact that it is up is no problem, since we know that gravity will be greater and will pull the pitch down, just as we observe. But the movement to the right is problematic, since that is definitely not what we observe. And that, in a nutshell, is our mystery.
So, what is going on here? Mike Fast posed this question to me in early May, 2011. Mike was the first one to recognize the unusual nature of this pitch, and he contacted me to ask whether I had an explanation for it.
In turn, I contacted a number of experts in the aerodynamics of sports balls, including my frequent collaborator Professor Rod Cross, a Rod did what really good physicists often do. He came up with some interesting experiments in an attempt to uncover what is going on with our mystery pitch, and I believe he has succeeded.
Rod has written a paper on his findings, “Aerodynamics in the classroom and at the ball park (PDF file),” that has been accepted in American Journal of Physics and will be published in the coming months. He also has a YouTube video, “How to Curve a Baseball or Swing a Cricket Ball,” that very nicely demonstrates the physics of what is going on. I highly recommend both the paper and the video.
In what follows, I will summarize Rod’s findings.
The key to understanding the movement on the Garcia pitch is a phenomenon that is responsible for the movement (in cricket it is called “swing”) of the cricket ball, which I will now explain with the help of the following cartoon.
 |
| Bird’s-eye view depicting the swing of a cricket ball. |
Consider air passing over the moving (but for the moment non-spinning, seamless, and smooth) spherical ball. A very thin layer of air (the “boundary layer”) actually sticks to the surface of the ball. Because of frictional forces, the air in the boundary layer slows down, stops, and separates from the ball, leaving a low-pressure wake behind the ball. The combination of high pressure in front of the ball due to the oncoming air and low pressure behind it leads to the drag force, which acts opposite to the direction of motion of the ball and slows it down.
In this case, the boundary layer separation is symmetric with respect to the direction of motion of the ball, and there are no transverse forces. For reasons discussed below, the separation point may shift upstream on one side and downstream on the opposite side of the ball.
Looking at the ball from the bowler’s viewpoint, we see that the separation point at the left side of the ball occurs further downstream than on the right side, resulting in a deflection of the airflow to the right. In effect, the ball exerts a force on the air to the right. Newton’s Third Law requires that the air exert an equal and opposite force on the ball, resulting in the swing of the ball to the left.
One way to break the symmetry of the boundary layer separation is to spin the ball. For example, if the ball in the picture were spinning counterclockwise (ignoring for now the seams and the roughness), the ball would pull the boundary layer along with it on the left side of the ball and push against it on the right side, resulting in separation occuring further downstream on the left than on the right. The resulting leftward force on the ball is the conventional Magnus effect.
Another way to break the symmetry occurs if there is an asymmetry in the surface roughness of the ball. Again consider the cricket ball in the figure and let us suppose that the ball is perfectly smooth except for the raised seam. Because of the orientation of the seam relative to the air flow, the left surface of the ball is rougher than the right surface. When air flows over a rough patch, such as a seam, the character of the flow changes from smooth (“laminar”) to turbulent.
The experts—and I am not one of them—say that when the boundary layer air is turbulent, it mixes with high-speed air adjacent to it, thereby increasing the average air speed in the boundary layer. The net result is that the air takes longer to separate from the surface.
Therefore, the boundary layer separation is delayed on the left side, resulting in a deflection of the air flow to the right and a corresponding deflection of the ball to the left. That force is responsible for the conventional swing of a cricket ball. In fact, a cricket bowler typically spins the ball about the equatorial seam, so that the orientation of the seam relative to the motion does not change as the ball rotates.
For a baseball, things are a bit more complicated because of the “figure-eight” seam pattern on the ball. Nevertheless, the same essential physics applies. Spinning balls have a Magnus effect. and an asymmetry in the surface roughness can result in transverse forces.
The most common example of the latter is the knuckleball. Since the ball is thrown with little or no spin, it is possible to orient the seams to produce an asymmetry in the roughness of the ball that persists throughout the trajectory. If the ball is spinning rapidly, as it is for most “normal” pitches, then conventional wisdom is that the effect of the seams is averaged out during the flight, resulting in no net transverse force. But conventional wisdom is not always right, as we shall see shortly.
Everything I just explained has been known for a long time. What is new are the very clever experiments of Rod Cross, as explained in his paper. I won’t discuss all his experiments but only the one relevant to the Garcia pitch.
Cross’s clever idea is to investigate the effect of seams on a polystyrene ball with approximately the same size as a baseball but much lighter. With the same size and seam pattern, the aerodynamic forces will be about the same as on a normal baseball. However, since the polystyrene ball is much lighter than a baseball, the deflection of the ball due to the forces will be larger and much easier to measure.
A sketch of the ball and seam pattern through one revolution is shown in the figure below, which I have taken directly from Cross’s paper (Fig. 11).
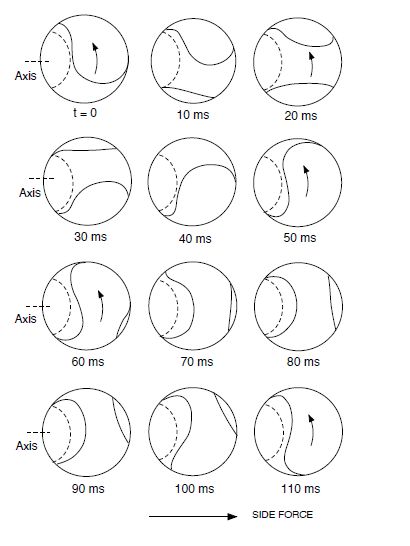 |
| Seam orientation during one revolution for one of Cross’s experiments. The ball is shown from the catcher’s viewpoint, and the spin axis is horizontal (backspin) and tilted slightly in the forward direction. Note the white patch shown by the dotted line on the left side of the ball that persists through one revolution. Thanks to Rod Cross for permission to use his picture. |
The ball is moving in a direction that is out of the plane of the page (catcher’s view) and is spinning about a horizontal axis (backspin) with the axis tilted slightly forward (i.e., there is a small “gyro” component to the spin). The figure shows clearly that there is a smooth white patch on the left-hand side of the ball that persists through a complete revolution. Therefore, averaged over one revolution, the left side of the ball is smoother than the right side.
The expectation is that the boundary layer separation will be shifted downstream on the right side, resulting in a side force to the catcher’s right. That is exactly what Cross sees in his experiment.
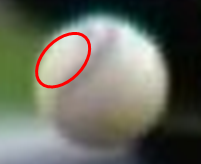 |
| Frame grab of the Garcia pitch, with the red oval indicating the location of the white patch. |
Now let’s return to the Garcia pitch. If you play back the video one frame at a time, you will see that the upper-left quadrant of the ball maintains a white patch throughout the trajectory. The spin axis must therefore pass roughly through the middle of the white patch (the “North Pole” of the ball).
It must also happen that the spin axis passes through another white patch diametrically opposite (the “South Pole”). We can’t see it on the video because it is on the side of the ball facing the catcher, who sees the white patch appear in the lower-left quadrant. Therefore, the oncoming air “sees” a left-right asymmetry in the average roughness of the ball. The left side (with the white patch) is smoother than the right side (with the seams).
By analogy with the swing of the cricket ball, the movement on the pitch should be to the catcher’s right, which is exactly what actually happens. At least qualitatively, the mystery is solved.
It is interesting to use the PITCHf/x trajectory to estimate the size of the various forces on the ball. The ball was released at 80.1 mph. All three components of acceleration are determined from the standard fit to the trajectory. By counting revolutions, I estimate the ball is rotating at the rate of 800 rpm.
From that, I can use the experimental relationship between the rotation rate and the Magnus force to estimate the magnitude of the latter. Since the movement is determined from the components of acceleration, and since the Magnus contribution can be estimated, the additional acceleration due to the roughness asymmetry can also be estimated.
Here is what I find for the forces, all normalized to the weight of the ball:
- gravity = 1.00
- drag = 0.78
- Magnus = 0.32
- roughness = 0.63
Finally, let me remark that another way to have an asymmetry in the surface roughness is to scuff the ball and arrange for the spin axis to pass through the center of the scuff. Then the side of the ball with the scuff always has the same orientation relative to the air flow, resulting in an asymmetry in the roughness.
Which way the ball deflects will depend on the relative importance of the scuff and the seams. If rotating the ball rapidly results in no net asymmetry due to the seams (the “conventional wisdom” referred to earlier), then the ball will deflect in the direction of the scuff.
oooo!
Speaking as a Brit and a baseball and a cricket fan, can I just say… ooo, NEAT 😀
Didn’t think that was possible with a baseball!
And if you think THAT was funky, check out http://en.wikipedia.org/wiki/Reverse_swing – a phenomenon which has been confusing all and sundry in cricket for ages.
Nice article Alan. My experience is that it is exactly this effect that is used to throw a cutter. It is extremely to generate the spin angle needed to throw a fastball that does not move laterally in the direction of the pitchers throwing hand. A different force than the traditional magnus force is needed to account for the glove-hand lateral movement.
Ike…that is a *very interesting comment* about the cutter. I need to look at some slo-mo of Mariano’s pitches to see what I can learn. Just when I thought I was done with this project….
Now that I reread.my comment, I meant to say “extremely difficult”, and somehow the word difficult just didn’t get typed.
I tried to do a quick google search for Mo-slo-mo, bit didn’t find anything from the CF angle before I posted. Anyway, to get the cutter effect from magnus alone, you’d have to angle your hand in a way that it doesn’t normally want to go, and I have a hard time thinking that doing so wouldn’t lead to injury.
Ike…the paper you are referring to may be Ref. 15 of Cross. Lloyd Smith has a portable TrackMan device that he is using for studies of baseball aerodynamics. Ref. 15 is a conference proceeding from 2010 in which Cd and Cl were measured for a variety of different baseballs and softballs. So, Rod was a bit careless with his pronouncement.
Last summer, Lloyd Smith and I discussed doing measurements on real baseballs much like the ones that Cross did on lightweight balls. We were going to project the ball at realistic speeds, then use Trackman to measure the trajectory and a high speed camera to get the spin rate and axis. We never found the time to do it—perhaps sometime this year.
Now that I have some time to read Rod Cross’s paper, I have (so far) one nitpicky point to make on it. On pg3, he says (and I’m going from memory because I’m writing on my phone) ‘previously the raised stitching has been shown to affect the flight of the knuckle ball, but has not been shown to affect amy other pitches’. This is only partially true. As many players know, and one study I read 2 or 3 years ago, (whose authors I really wish I could remember right now) found, the height of the seams do affect both Cl and Cd of ‘normal pitches’. Most players know this because the balls used in HS, college and professional levels all have different seam heights.
“Since the spin axis is tilted, there is reduced backspin (so that the ball drops more than a typical four-seamer).”
—-That is an incorrect cause-effect statement. A standard 2-seam fastball, thrown with the same exact tilt of the spin axis, would have a very much higher rate of backspin. The splitter has a low rate of backspin because the index and middle fingers are “split”, rather than directly behind the ball, therefore they cannot apply as much spin-inducing force to the ball. (Think of the extreme example of the ball being launched from a grip in which the only contact was exactly at the north and south poles…if it could be done, this would be another way to relase a ball with no spin at all).
I have not read Cross’s paper yet, but from your summary, I am left still puzzled. With any spin rate at all, the asymmetry of a 2-seam pitch, or this splitter, must be “spin averaged” to little or no asymmetry because the seams are actually symmetric with respect to the spin axis.
A scuff ball specifically takes advantage of the fact that a scuff on one of the poles of the spin axis cannot be averaged out by the ball’s spin.
There are some pitch grips that can give seam patterns that are not “averaged out” with spin, but the Garcia pitch dopes not look like one of those at all.
Rabi Mehta’s fascinating analysis of cricket ball swing types suggests that boundary-layer turbulence-induced trends are highly velocity dependent—astoundingly, he shows convincingly that cricket balls released from identical grips may give exactly opposite senses of movemenet (swing) depending only on the bowler’s velocity. The unfortunate bowler with “transition” velocity may get no movement at all!
Again, I look forward to reading Cross, but I suspect that this mystery may not be solved until Mehta’s work is taken into consideration.
My statement, as written, causes some confusion. What I meant to say is that a typical 2S fastball (as opposed to a splitter) has its spin axis tilted relative to a 4S. I realize that a splitter, although thrown with a similar spin axis as a 2S, has considerably less spin. Sorry for the causing the confusion. I certainly agree with Lee’s statement.