On rotation order and win probabilities
Every Opening Day, I inevitably see someone propose the idea of an unconventional starting rotation order, in an attempt to tilt the odds a small amount. The basic idea goes a little like this—punt Opening Day by letting your team’s fifth starter sacrifice himself, facing the opponent’s number one. You then gain a pitching advantage over the next few starts, by having your number one face their number two, your two against their three, and so on and so forth. Given two identical teams and a five-game series, it seems to make some sort of intuitive sense.
It seems obvious that among off days, rainouts and injuries, any possible advantage won’t last very long before the schedule is a jumbled mess. But is it actually true? Is the advantage significant in any form? Why not crunch some numbers and find out?
I’m going to start with a simplistic model using easily digestible numbers, and then I’ll fold real world data into what we’ve got.
In theory
To keep it simple, let’s make the very, very generous assumption that every team has five identical perpetually healthy starters who rotate in a perfect cycle. If the schedule consists of 162 consecutive days, every team’s ace will pitch on day one, day six, day 11, and so on, all the way through to game 161. Expressed graphically, this looks a little something like this. Each row is assigned to a single team, while the columns correspond to days on the calendar. The days where each team’s day one starter is scheduled to throw are marked in red.
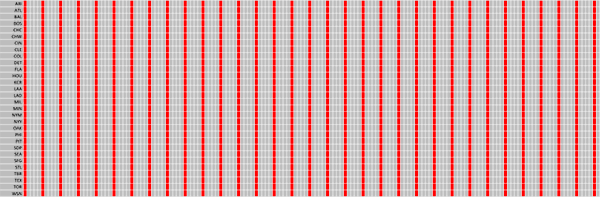
Again, using simple numbers (and a little help from Chris Jaffe), let’s spitball the quality of each rotation spot so that first, second, third, fourth and fifth starters for every team have ERA+s of 125, 110, 100, 90 and 80, respectively. Naturally, a conventionally ordered pitching rotation would result in win probabilities of 50.0 percent for every game, since your 125 ERA+ pitcher is facing their 125 ERA+ pitcher, etc.
A staggered rotation, in this case, would mean punting Opening Day by throwing an 80 ERA+ pitcher against a 125 ERA+ ace. Now, it’s not a complete forfeit—there’s some non-zero chance that your fifth starter will manage a minor miracle and beat the opponent’s number one. It’s actually a fairly simple calculation by using the Pythagorean expectation formula, and it turns out that an 80 ERA+ pitcher has a 29.0 percent chance of beating a 125 ERA+ ace, given perfectly equal offenses. Not great odds, but it’s clearly not zero.
It’s also true that pitting your number one against the other team’s number two results in a win probability that’s higher than usual. Again, using the Pythagorean expectation formula in this purely theoretical vacuum, it turns out that you get somewhere around a five to six percent win probability boost above the usual 50 percent over the next four days each.
The total increase in win probability over a full five-game turn of the rotation? 0.3 percent. Seriously. A team’s win probability, when facing a perfectly symmetrical opponent, goes from 50.0 percent to 50.3 percent when deploying this strategy. On average, a team would pick up one free win every other year when using a staggered rotation. In this theoretical vacuum. Not exactly a game changer.
In practice
Let’s break away from a perfect even 162-game schedule.
I plugged the full 2011 major league schedule into the model, keeping everything else the same. As before, I’ve marked every theoretical ace start in red. This one’s far more interesting, due to the off-days.

I simulated the 2011 season 30 times, one for each team’s attempt at a staggered rotation, while holding the rest of the league constant. The average win probability boost? 0.14 percent. The Brewers happened to have a schedule that gave them a half of a percent. Most were far less fortunate, and some actually took a tiny (tiny) probability hit. The strategy really, really doesn’t work.
But hey, we’re deep into this now, why not throw one more wrench into the model? How about actual pitchers?
I carried out the same exercise as before, this time replacing the theoretical pitchers with the pitchers who actually started every game in 2011. As a replacement for the ballpark ERA+ figures, I used their season FIP. As you might expect, probabilities varied quite a bit more here than in our theoretical vacuum. Even still, the average win probability change was a negative two percent.
It doesn’t work. Not in a five-game playoff series, not in a perfect theoretical season, and not using real game data.
References & Resources
- All schedule data from Retrosheet. FIP data for the actual pitchers from Fangraphs.
- The 2011 schedule doesn’t perfectly line up at the end, because the Dodgers and the Nationals ended up finishing the season with only 161 games.
- For the record, I used 2011, because 2012 had the extra wrinkle of the A’s and the Mariners opening the season in Tokyo a full week before everyone else had their opening days.

Dan,
Coincidentally, I made this suggestion over at Bucs Dugout this season (after watching A.J. Burnett pitch well but go 0-2 against Cubs/Dodgers* aces), and it got dismantled there too.
Good work.
*—Although it’s not exactly easy to tell who the Dodgers’ “ace” is.
My team doesn’t have an ace. Hell, it doesn’t have a 2 or a 3 pitcher. It has 3 3’s (maybe), a 4 and a 5.
Fascinating stuff, and great to see someone step up to the challenge of running the numbers.
I’m amazed it came out so low in the ideal staggered 5 game series. But it really illustrates the importance of never simply tossing a game from the onset.
Good research, Dan.
Perhaps it may sense when other factors are involved during the course of the season on a single game-to-game basis, such as when a lowly team could use their #5 or #1 and is facing a top team one night with their # 1 going but when that same lowly team is facing a mediocre team the next that has already used their ace. Or when injuries to position players are involved.
But as you pointed out there’s no point in avoided all #1 vs #1 matchups from the outset.