Player Value: The Last Piece of the Puzzle
There is no simple answer to the question of how much a player is worth. A common, even if somewhat flawed, approach is to compare the salary of similar players, creating a set of “comparables” as if it were a real estate transaction. The problem with this approach is that it assumes there is some intrinsic value to a player who can generate six wins.
As a six-win closer, Billy Wagner’s “value”—the marginal revenue he generates for his team (relative to a replacement player)—can range from less than $1 million to more than $10 million, depending on his employer. In the rational world of economics and finance, a player’s dollar value is largely situational; it depends on the market, as well as on the team’s level of competitiveness.
The economics of winning dictate that a team’s level of competitiveness has a dramatic impact on a player’s value. There is little glory or marginal revenue from improving a 69-win team by six wins. The revenue gains from improving a 79-win team by six wins are somewhat larger, but they pale in comparison to improving an 89-win team by six wins. In the latter case, the player acquisition that generates the six-win improvement is considered the “last piece of the puzzle.”
This could be exactly what the Mets were thinking (and calculating) when they settled on their deal to bring Billy Wagner to New York. If you entertain the assumption that Omar Minaya’s offseason moves prior to the signing of Wagner elevated the Mets to, say, an 89-win team, Wagner becomes the potential “last piece of the puzzle” for the Mets to secure a berth in the postseason. Improving an 89-win team to 95 wins more than doubles the team’s chances of making the playoffs (93% vs. 44%).
Crediting Wagner and his expected six-win performance with the postseason revenue impact of thrusting his team into the playoffs brings his annual value to over $10 million. Figure 1 shows Wagner’s value to the Mets in three different scenarios: as a 74, 82 and 89-win team, prior to his arrival.
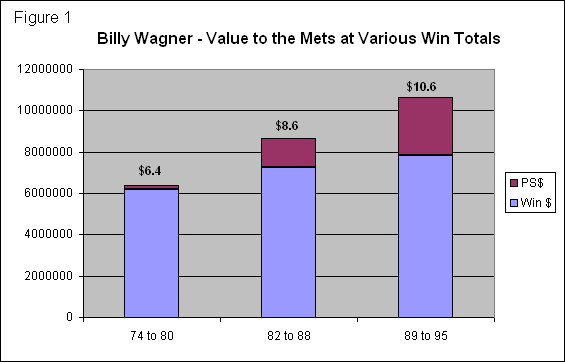
The “step-up” in value from each scenario is over $2 million. The Win Dollars (Win $) represent the incremental revenue that a player generates from improving his team by six wins (in the case of Wagner) and the resulting attendance, concessions and other revenue. The Postseason Dollars (PS $) represent the change in the expected value of the postseason revenue stream, based on how the player’s performance increases his team’s odds of reaching postseason paydirt. (For more on the methodology of calculating a player’s value see my article Player Value: The Postseason Effect.)
Conversely, if Wagner were the property of a “competitively challenged” 75-win Pittsburgh Pirates team, his value would be only $2.2 million. However, even a “small-market” team like the Pirates could justify paying $6.2 million for Wagner if he is their last piece of the puzzle, joining a hypothetical 89-win Pirates team and improving them to 95 wins.
There are three key leverage points in the player value equation: the player’s level of performance, the team/market and the competitiveness of the team. In order to understand the relative importance of each, I developed an example to allow a comparison. Regarding playing performance, I will compare Alex Rodriguez, a 10-WARP Yankee in 2005, and Brandon Inge, a six-WARP Tiger in 2005. (I’ve rounded their WARP values from the bp.com website to the nearest whole number to simplify the math for my illustration.)
For a team comparison I’ve chosen the mega-market Yankees, who earned about $400 million in revenue in ’05, vs. the Tigers, who generated about $100 million in revenue prior to any revenue-sharing distribution by the league. To demonstrate the power of the situation (i.e., the team’s level of competitiveness), I used my model’s estimates of marginal revenue at various win levels.
The first scenario looks at A-Rod as a 2005 Yankee and Inge as a 2005 Tiger. The 95-win Yankees’ revenues are $22.1 million higher, due to the on-field contribution of A-Rod, while the 71-win Tigers benefited by $1.6 million from Inge’s six-win contribution in ’05.
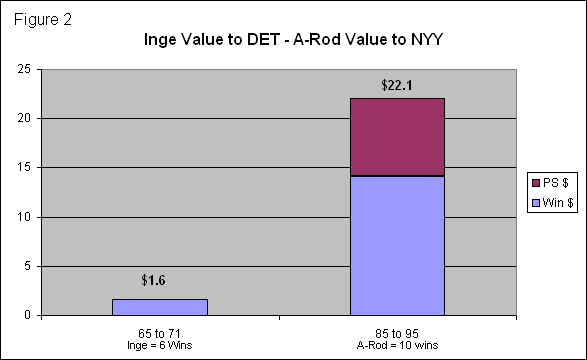
The dramatic difference in value has much to do with the win total of the two teams. The Yankees’ 95 wins placed them in the thick of the playoff hunt. About $8 million of A-Rod’s $22.1 million in value comes from revenue generated from the Yankees reaching the postseason, as A-Rod’s on-field contribution significantly elevates New York’s chances of reaching the playoffs. (Presently, the Yankees’ Net Present Value of reaching the playoffs are actually lower than the MLB average, due to the diminishing returns from reaching the postseason for 11 consecutive years.)
The limiting factor in Inge’s value is the win level of Detroit. Inge’s on-field contribution generates six wins that are simply not as “important,” as measured by fans’ responsiveness to changes in a team’s win total. Attendance and other revenues do not increase much if a team improves to 71 wins vs. 65 wins.
Let’s look at how values might change if we switched A-Rod and Inge. As a Tiger, A-Rod would become a 10-win third baseman on a 75-win team, and Inge would be a six-win player on a 91-win Yankee team. With the switch of teams, Inge’s value of $13.2 million is more than three times greater than A-Rod’s $4.2 million. (See Figure 3.)

This comparison leads to the question: “Is it the team—the mega-market Yankees—that drives value, or is it the win total and playoff contention?” To illustrate the power of the win total, let’s look at the value of a six-win player across several teams (Yankees, Tigers, Twins and A’s) at various win totals. Figure 4 shows how the value of a six-win player increases as a team’s win total increases and it moves into contention for the postseason and its hefty revenue stream. (Note: “85 wins” refers to the value of a six-win player added to a 79-win team).
The chart clearly shows that there is a team or market effect—contrasting the Yankees with the Twins or A’s—particularly at 85 wins or more. However, the larger effect on player value may be the team’s level of competitiveness. As each team moves up the win curve, its revenues accelerate, peaking at about 95 to 98 wins.
There are several interesting conclusions shown in Figure 4. Note how the Yankees’ economic advantage over small-market teams diminishes at lower win totals. As an 80-win ballclub, the Yanks hardly maintain any true economic advantage over Detroit or Minnesota in bidding for a six-win player. Also, a Tiger team in playoff contention (85 to 90 wins) will generate more revenue by adding a six-win player than an 80-win Yankee team. Even a contending Minnesota or Oakland ball club has as much economic justification to pay $6 million per year for a six-win player as a sub-.500 Yankee team.
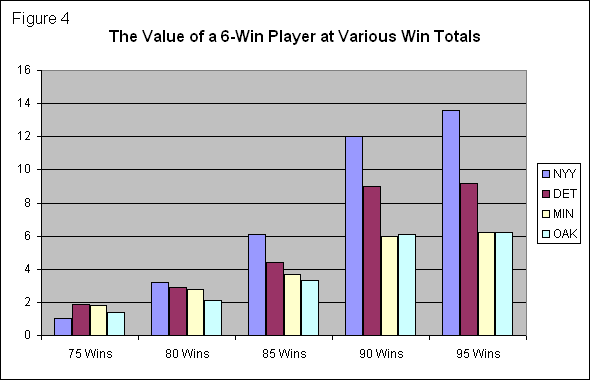
While market size is an important variable in determining a player’s value, a team’s location on the win curve is also a significant factor. For the Twins, a six-win player’s value doubles for a 95-win team vs. an 80-win team, while for the A’s, his value would triple. In the ultra-competitive free-agent market, pricing tends to be set by teams adding the last puzzle piece—or at least teams that think they are adding the last piece to vault them into the postseason.
With the Blue Jays’ signing of BJ Ryan and AJ Burnett, one can only assume that General Manager JP Ricciardi believes these players will be the difference between mediocrity and a playoff berth. Even if he’s correct, for these contracts to “pay out,” the Jays would need to reach the postseason three times and raise ticket prices by 20% over the five-year life of these two contracts. To protect the Jays on the downside, Ricciardi likely believes he will be able to unload Ryan to the Yankees (or another team grasping for the postseason) if his team turns non-competitive.
This analysis attempts to illustrate that player value is dynamic, not static. A player’s value is dependent on his performance, his team and their market and the team’s level of competitiveness. There may not be a simple answer to the question of how much a player is worth, but there is an answer for every player on any team at every win level.
