The drama index
The other day, I wrote about something I called the Season Leverage Index. The idea is simple: I want to quantify how much more important victories in September are compared to victories in April.
After the fact, of course, they’re not more important at all. A win is a win, no matter when it occurs. But as the season is played, and the games dwindle down to a precious few, each game a contending team plays certainly feels more important. Books are written about the final contests of a season, not the first ones. Think we ever would have heard of Merkle’s Boner if Fred Merkle hadn’t touched second base in May? Or that we ever would have heard Bobby Thomson’s Shot Heard ‘Round the World if it had been smacked in the spring? It would have been more like a shot heard ’round the borough.
Yet I’m not really satisfied with this nebulous feeling that late-season games are more important than early-season games. As I said, I don’t think they’re really more “important.” I think they’re more “dramatic.” And I like drama as much as the next theater critic. There’s nothing wrong with enjoying (and writing about) those end-of-season nail-biting battles.
Secondly, being an unmitigated baseball nerd, I want to know “how much more dramatic.” Seriously. If September games are more dramatic than April games—so much that we even vote for our MVPs based on how well they did in the more dramatic contests—how much more dramatic are they? Or, what’s the potential for them to be dramatic? (In the end, players provide the drama; the timing of those games just provides the context.)
That’s what I started to do in my last article, with my notion of season leverage index. But I’ve had second thoughts about the name. We’re not really talking about leverage here, we’re talking about drama. So I’ve got a new name for my concept: the drama index. There is no guarantee I won’t change the name again next week.
The idea is simple, but the spreadsheet is ginormous. To calculate the drama index, I assume that each team has a natural distribution of games it is likely to win in the remaining season. I do this by calculating a binomial distribution (BINOMDIST in your Excel spreadsheet) for each possible situation during the season. For instance, the binomial distribution of a .500 team with ten games to go will project the following number of wins this percent of the time:
Games Percent
0 0%
1 1%
2 4%
3 12%
4 21%
5 25%
6 21%
7 12%
8 4%
9 1%
10 0%
So if this team has to win seven or more of its ten remaining games to make the postseason, there is a 17% chance they will do so (count from the bottom up: 1% plus 4% plus 12%). That’s called cumulative probability.
Next, I look at what would happen to the binomial distribution if a team were to win or lose that day’s game. For instance, if our example team were to win its game, it would have to win six of its next nine games to qualify for the postseason (momentarily setting aside its competitors’ outcomes). The cumulative probability of winning six of nine is 25%. But if it were to lose that day’s game, it would have to win 7 of its next nine games and the cumulative probability of that is 9%. So, the drama index is 16 points (25% minus 9%). That’s the potential impact of that day’s game on the team’s chances of making the postseason.
My recipe for the whole shebang: look at each day of the season and calculate the number of wins each team is behind the nearest postseason position (in other words, its division leader or the wild card leader, if closer) or, for division and wild card leaders, how much they are ahead; then use the binomial distribution to calculate the impact of a win or loss on its chances of reaching the postseason (assuming the competition plays .500 ball the rest of the season. Since the index is updated daily, .500 isn’t a prediction. It’s just a guideline for the index.)
In the above example, the highest drama index goes to the team that has to win five or six of its remaining ten games, because the impact of a win or loss in those two situations gives them the same result (25 points). I’m not going to show you the math. Trust me.
For technical reasons I’ll describe later, I used a .550 winning percentage in my binomial calculations instead of .500. But .500 is most useful for the explaining. Oh, and one last note: I divided each team’s drama index by the average drama index of a .500 team throughout the year. So a drama index of 1.0 is a useful benchmark.
Enough with the details. I’ll give more details below, and I’m happy to answer any questions in the comments. But let’s look at some particular teams. Here is a graph of the day-by-day drama index of the Washington Nationals:
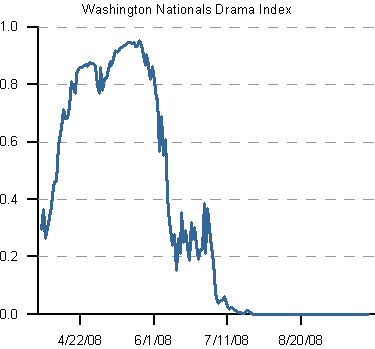
In late May, the Nats were “only” eight games out of first, and there was a little sizzle going on. But overall there was very little drama to the Nationals’ season, and none at all after mid-July. In fact, every single one of their regular-season games had a drama index under one.
There were six teams that didn’t have a single game with a drama index of at least one. They were:
{exp:list_maker}Chicago Cubs
Kansas City Royals
Los Angeles Angels of Anaheim
Seattle Mariners
Tampa Bay Rays
Washington Nationals{/exp:list_maker}See how it works? The Nationals had no drama cause they were never really competitive. The Angels had no drama cause they were too good and the competition was too weak. The Rays didn’t have any drama in this system because, even though they battled the Red Sox for first place in the AL East, they had the wild card qualification to fall back on. Their postseason appearance was never seriously at stake.
By the way, several people mentioned playoff probabilities (such as those at Baseball Prospectus) as a way of measuring game-to-game drama. However, playoff probability is different. If two teams are tied for the division lead late in the season, they’re playing very dramatic games—but if they both win, their playoff probabilities will hardly change. The analogy is imperfect, but playoff probabilities are like WPA; they reflect the outcome. The drama index is similar to Leverage Index; it reflects the importance of the game, regardless of the outcome.
Back to the matter at hand. The following teams had the most games with a drama index greater than 1.0:
{exp:list_maker}Minnesota Twins: 68
Los Angeles Dodgers: 66
New York Yankees: 64
New York Mets: 59{/exp:list_maker}You’re probably not surprised by this list, though I was a bit surprised that the Yankees were in the top four. Here is a graph of their day-to-day drama index:
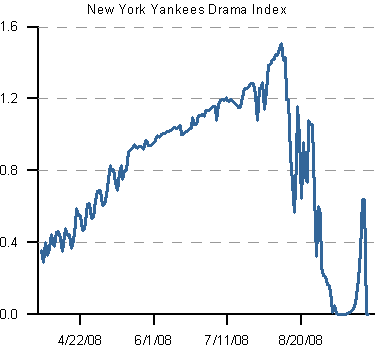
The Bronx Bombers were fighting in June and July, and in late July they had almost tied the Red Sox for second place (and the wild card lead). The potential for theatrics was high. But Boston surged ahead and the Yankees couldn’t maintain the pace. By early September, their DI was pretty much zero, though there was a little sizzle around September 22 when they hadn’t been eliminated yet. Once they were, their index dropped to absolute zero.
The Mets, on the other hand, kept the drama going until it spiked at the very end:
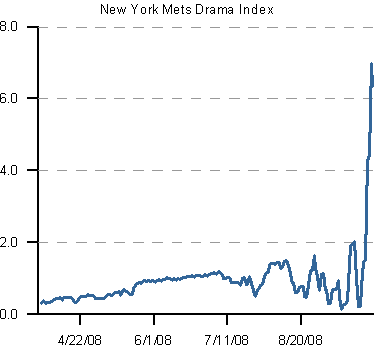
There were some wild swings to the Mets’ late season, as they battled first the surging Phillies for the NL East lead, then the surging Brewers for the wild card lead. Their last two games were particularly dramatic; the next-to-last game actually had a slightly higher index than the last game. When they won and the Brewers lost, the DI for the final game eased a bit.
The Dodgers also had a lot of ups and downs to their season, though not as dramatic as the Mets’:
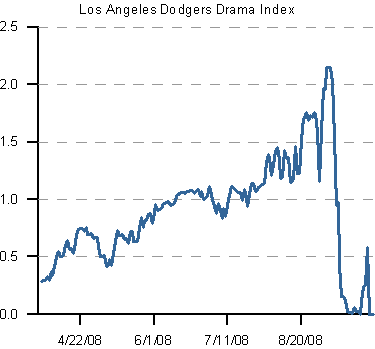
August was the month of drama at Chavez Ravine, when the Dodgers surged to catch the Diamondbacks. Their index spiked over 2.0 just before they finally caught Arizona in early September, and it fell quickly as they pulled away. There was a little surge of drama at the end, when the Diamondbacks played a little better and the Dodgers played .500 ball. But once they clinched the title, the index fell to zero.
But, of course, nobody could beat the American League Central for drama, where the White Sox and Twins extended the season for two extra days of excitement. Let’s talk about the Sox:
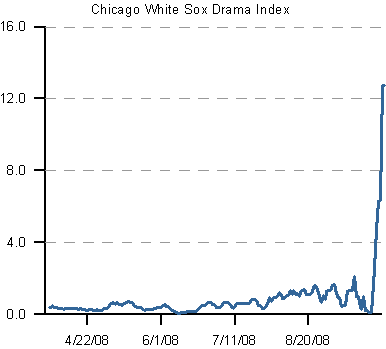
That is one wacky graph. The Sox only had one game with a drama index above 1.7 before the final Wednesday of the season, then they played nothing but thrillers. It was mostly their own fault, as they lost five straight games before winning their final three.
See that little extension to the right at the very tippy top of the graph? That’s because the Sox had two do-or-die games in a row (against the Tigers and Twins), with the maximum drama impact of 12.7. That’s the way the system works. Games at the end of the pennant race are nearly thirteen times as dramatic as the benchmark, and about 40 times more dramatic than games at the beginning of the season.
As sort of a postscript, here is a little information for you to nosh on: the record of all teams in games with a drama index greater than one. For each team, you’ve got the number of games of high drama (over 1.0), the team’s overall average drama index, and their record in games over 1.0. The best teams when the games “counted” were the Brewers, with a winning percentage of .708, and the Phillies, at .700.
Team G>1 Avg. W L Win% MIN 68 1.109 41 27 .603 LAN 66 0.867 37 29 .561 NYA 64 0.763 36 28 .563 NYN 59 0.916 35 24 .593 FLA 40 0.632 21 19 .525 PHI 40 0.778 28 12 .700 DET 39 0.631 21 18 .538 TEX 39 0.641 18 21 .462 CHA 35 0.831 22 13 .629 STL 33 0.594 16 17 .485 ATL 31 0.538 10 21 .323 BAL 29 0.474 15 14 .517 OAK 28 0.486 13 15 .464 MIL 24 0.819 17 7 .708 SF 22 0.554 8 14 .364 ARI 16 0.490 7 9 .438 HOU 13 0.592 4 9 .308 CLE 9 0.389 3 6 .333 TOR 9 0.537 3 6 .333 SD 6 0.419 1 5 .167 PIT 5 0.491 0 5 .000 CIN 4 0.490 0 4 .000 COL 4 0.579 2 2 .500 BOS 1 0.323 1 0 1.000 CHN 0 0.169 0 0 .000 KC 0 0.358 0 0 .000 LAA 0 0.150 0 0 .000 SEA 0 0.245 0 0 .000 TB 0 0.239 0 0 .000 WAS 0 0.334 0 0 .000
Think those records contributed to the fine MVP showings of the Ryan’s? (Howard and Braun). That will be the subject of my next article.
References & Resources
Here’s a little information about the team-by-team (and game-by-game) drama index in 2008:
{exp:list_maker}Last year, the average drama index for each team was .546. About 6% of all team games had a drama index of absolute zero (By that, I mean that one of the two teams in the game had a DI of zero.).
When a team has to win its one remaining game to make the playoffs, the drama index is 12.73. That applied to only a few team games last year: the White Sox/Tigers and the White Sox/Twins games, after the regularly scheduled season had ended.
Games in which there is a tie for first going into the last game of the season, such as those played by the Mets and Brewers last year, have a drama index of 6.36. The index is lower than that of the one-game playoff because there is a 50% chance that the other team will lose its game.
Games in which the team has to win two of its last two games, such as the Mets’ next-to-last game, have a DI of 7.0. At that point, the Mets were a game behind the Brewers, and the index assumes Milwaukee would win one of its two remaining games. Therefore, the Mets would have to win two to force a playoff.{/exp:list_maker}The drama index is very steep. As the season closes in late September, most competing teams will have a game DI of 2.0 to 4.0; only in the last four or five games is the index likely to exceed 4.0.
Why did I use binomial distribution tables for a probability of .550 instead of .500? Consider the following cumulative probability table for a .500 team:
Games Left
Wins Needed 0 1 2
0 1.00 1.00 1.00
1 0.50 0.75
2 0.25
Now, consider the team that has to win two of its next two games (which is 0.25 on the table). The drama index of that situation is .5 (if you move to the left on the table, you can hopefully see why; it’s .5 minus 0). Now look at the team that has to win one of its next two games (0.75 on the table). Move to the left, and the drama index is also .5 (1.0 minus .5).
I don’t know about you, but this table doesn’t pass my smell test. I think that having to win two of the next two games is more dramatic than having to win one of the next two. I think the problem is related to the way the table deals with situations at the “ends” of the distribution.
To fix it, I changed the underlying probability to .550, which is the winning percentage of a 90-win team (which usually qualifies for the postseason). The table now reads:
Games Left
Wins Needed 0 1 2
0 1.0000 1.0000 1.0000
1 0.5500 0.7975
2 0.3025
Now, the drama index for a team that has to win two of its next two games is .55. For the team that has to win one of its next two, it’s .45. I have no idea if that’s “right,” but at least it passes my smell test.
